The elusive iforceahedron
OK, so now we are going to try to force a tensegrity structure to take on the shape of a truly regular icosahedron. But we don’t have to search blindly for a way; we are armed with the results of this MathStream post about how to do it.
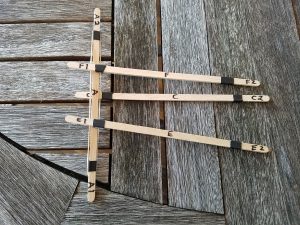
To sum those results up, if we label the ends of the struts as shown in this diagram,
then we need to place twelve rubber bands along the following paths: A1-C1-A2-F1-E1 A1-B1-A2-F2-E2 D1-B2-D2-F2-E2 D1-C2-D2-F1-E1 B1-E1-B2-D2-A2 B1-F1-B2-D1-A1 C1-F2-C2-D1-A1 C1-E2-C2-D2-A2 E1-A1-E2-C2-B2 E1-D1-E2-C1-B1 F1-D2-F2-C1-B1 and F1-A2-F2-C2-B2.
| Materials |
|---|
| 6 labeled struts |
| 12 rubber bands |
 |
These building directions are not as intuitive as the ones for the original not-quite-icosahedron, so I strongly recommend that you take your six struts and label the ends clearly before you start to build anything.
The first rubber band is easy: just take the ends of the struts named and space them out equally along the band, as shown below. Note this means that you need to leave more space on one side of strut A than the other, because there is only one connection between A1 and A2 on one side (namely C1) but two connections on the other side (F1 and E1).
The second rubber band is trickier: you have to make sure to leave the shorter segment of the band on the opposite side of strut A as last time, and stretch the longer segment around to connect to the far end of the E and F struts.
Before inserting the third or fourth rubber bands, it’s helpful to place the D strut among the others in roughly the position it will end up.
Here it is with the third rubber band (which really doesn’t yet want to stay in place).
And here it is after four rubber bands, which makes everything much more snug and gives the nascent structure much more shape.
At this point, it becomes much easier to simply wrap each rubber band around the five points it is supposed to connect, trying to keep an even amount of tension on the rubber as you go. Here’s the structure after two more rubber bands (the two that each intersect both ends of the B strut) — we’re half done!
The following two pictures show the state after eight and ten rubber bands, respectively.
And here we are upon first getting all twelve rubber bands in place. It doesn’t look regular yet, but maybe it just needs tweaking the rubber bands to make it symmetrical.
However, here we are after quite some time spent pulling rubber into the segments between the ends of parallel struts, to try to lengthen those segments and shorten the other segments. At this point, the rubber bands are getting pretty loose between the parallel struts, and taut to seem near the point of breaking on the other segments.
So we reach the inescapable conclusion that you can’t force a tensegrity structure on six struts like this to adopt the shape of a regular icosahedron. Is that a disappointing result? Quite the contrary; it’s always kind of exciting when things don’t work out the way you expect. It often means that something deeper is going on, waiting to be unraveled. And in retrospect, we had a clue from the original structure that this might not work out. Namely, you can observe in that structure that the ends of parallel struts are closer together than the ends of non-parallel struts, even though there are no direct rubber-band connections between these points. Intuitively, adding rubber connecting the ends of parallel struts can’t make them move any further apart, only bring them closer together. Hence, since we needed to spread those points apart to change the original tensegrity structure into a regular icosahedron, we were doomed to fail.
Thus, there remain mysteries in even the most familiar of tensegrity structures, that Studio Infinity will return to one day. Is there any way to create a truly regular icosahedron with tensegrity?












Thanks for this! You gave a great idea for a new kite design. Would you by any chance know the ratio of the length of the ribs versus the length of the struts?
Bart, thanks for a great question. If the structure actually came out to be a regular icosahedron, it would be easy to figure out the ratio: the struts are just the diagonals of regular pentagons made up of five of the sides of the icosahedron, so the struts would be (1+√5)/2 times as long as the elastic edges of the icosahedron. (That’s the golden ratio, approximately 1.618.)
In practice, though, the parallel struts move closer together, so they will be somewhat more than 1.618 times as long as the separation between parallel struts, and somewhat less than 1.618 times as long as the other connections between struts (which should all be the same length by symmetry). Unfortunately, I don’t know how to calculate what these exact separations should be — if I did, I’d have a better chance of designing a perfectly icosahedral tensegrity.