Anticos

Judging from at least one of the previous projects, Studio Infinity is intrigued with connecting polyhedra edge-to-edge. (Of course, connecting them face-to-face is interesting, too, but that’s pretty familiar from Legos and such; and vertex-to-vertex is the same as connecting dual polyhedra face-to-face.)

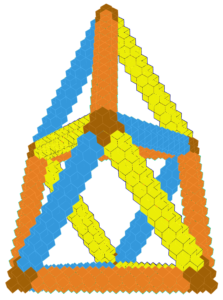
As you can see in the “blueprint” for the Boxtahedral Tower at right, connecting solids in this way often highlights symmetries that might otherwise be overlooked. In that case, edge-to-edge connections illuminated the threefold symmetries of cubes.

Even more surprising is that the most spherical of the Platonic solids, the icosahedron, can nestle into a cube with six of its edges just brushing the faces of the cube:

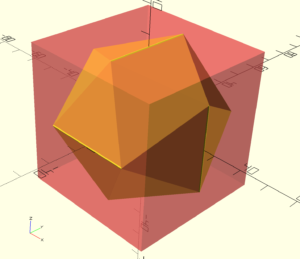
That means that if we connect icosahedra edge-to-edge, we should be able to make rectilinear structures, like with ordinary building blocks:

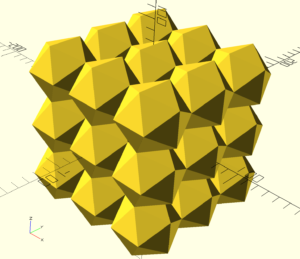
I wanted to find a way to show what was going on in this structure; but it seems as though the individual icosahedra get in the way of seeing the overall picture. You can’t see many of the actual edge connections at once.

Sometimes, however, what’s not there is even more illuminating than what is. Thus, the “Anticos” emerged: the negative space of the above configuration.

(More precisely, I enlarge each icosahedron by about 35% before carving it out of its respective cube. That step creates the “windows” in the sides of the cubes that lets you see the internal structure — without the enlargement, there would just be an infinitesimally narrow slit in each surface, so you would see nothing but a stack of cubes.)

Although usually S∞ focuses on structures that you can build by hand with ordinary materials, for something this intricate and detailed 3D printing seemed to be the only reasonable route to get a physical example in a reasonable amount of time. So here is the OpenSCAD source file and the resulting STL file. Since all of Studio Infinity’s content is Creative Commons-licensed, feel free to modify however you may like, and print if you have access to a 3D printer.

Now the icosahedra are revealed to embed in a lacy network of octahedra connected by rhombic prisms:

Although after the fact it may not be a mathematical surprise that octahedra show up between the icosahedra, it’s still visually striking, and I’d say that creating the physical model heightens our appreciation of how they all fit together. Moreover, physical models can reveal other surprising aspects of the underlying geometry. For example, this half-height prototype of the Anticos reveals a lovely network of hexagonal cross-sections of the packed icosahedra:

Hopefully, S∞ will have much more to say about connecting polyhedra both face-to-face and edge-to-edge in the weeks to come.