Octahedron Too
So when I was doing the math for the styrofoam-dowel tetrahedron, I noticed that locating the holes necessary for making a regular octahedron should be easy too. Naturally, having done the theory, it’s hard to resist actually building the thing.
The materials are exactly the same as for the tetrahedron, just the quantities are different. In fact, you can re-use two holes from each of the balls you made for the tetrahedron.
| Materials | Tools |
|---|---|
| 12 3/16″ dowels | thumbtacks |
| 6 4″ closed-cell styrofoam balls | thread, wire, or thin twist-tie |
| optional markers | ruler |
| drill with 9/64″ bit |
Then you have to measure out two different lengths of thread or twist tie, tied from one shaft of a thumbtack to another. The first is 2.1 inches, just like before; that’s the length of the sides of the square of holes we’re going to drill. The second is 3.15 inches, for the diagonal of the square. (If that value is ringing bells in your brain, you are absolutely right: if you chase down all of the math that went into this, we’re going for exactly π inches from pin to pin here, but the accuracy of this process is way below 1/100 of an inch, so even 3 1/8″ is good enough, for example.)
For each ball, you start with two arbitrary holes separated by the shorter distance (which can be previously used holes). Then you scratch (appropriate arcs of) circles of each of the two radii from each of those two holes. They should intersect in pairs (the closer arc to one hole with the farther arc from the other) to create four points for drilling arranged in a square on the surface of the sphere. Here’s a picture of the two thumbtack setups of the two different lengths, and one ball with the scratches in place.
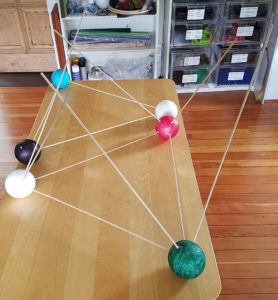
Once you’ve drilled all four holes in each of the balls (less any holes you might be re-using), it’s time to assemble. I recommend making two triangles first (one pictured on the right), ensuring that all pairs of unused holes point upward. Then use the remaining six dowels to insert in the remaining holes of one of the triangles, leaving six free ends up in the air.
Finally, flip over the other triangle and connect it as the top face, producing the final octahedron:
Theoretically, exactly the same procedures could be employed to make a section of the oct-tet lattice, but that would require a lot of scratching and drilling…