Arches and Sangaku in Memorial Hall
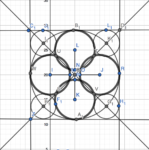
Housing the largest undergraduate dining hall, a lecture hall, a student bar, classrooms, music rooms and more, Mem Hall is almost always bustling with student activity. Unlike other buildings on Harvard’s campus however, the architectural style of Annenberg Hall is unique, a fusion of New England brick with gothic arches, cathedral-like stained glass, and detail-rich ornamentation. Although a mathematical perspective can shed insight on many facets of the building, two areas of particular interest can be seen housed within the ceiling structure of the 9000-square-foot great hall.
Read More
