Skeletal Polyhedra from Polyhedra: Part 1

After more or less independently stumbling upon the ideas for DodecaRT and TOWARD, a natural question is what polyhedra, in general, have skeletons that can be built using other polyhedra as vertex and edge components? Is there a way to generate families of new examples?

As a simple start, there is of course

(1) A cube of cubes:

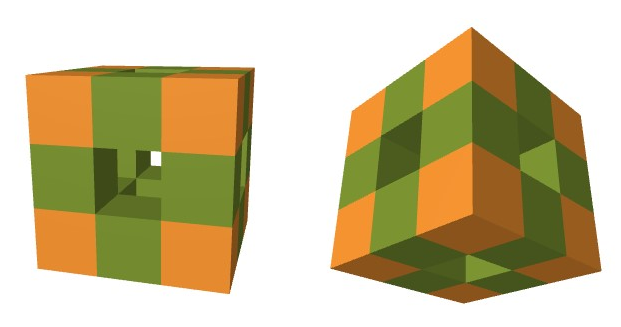
And at this point we had also seen:

(2) A rhombic triacontahedron of regular dodecahedra

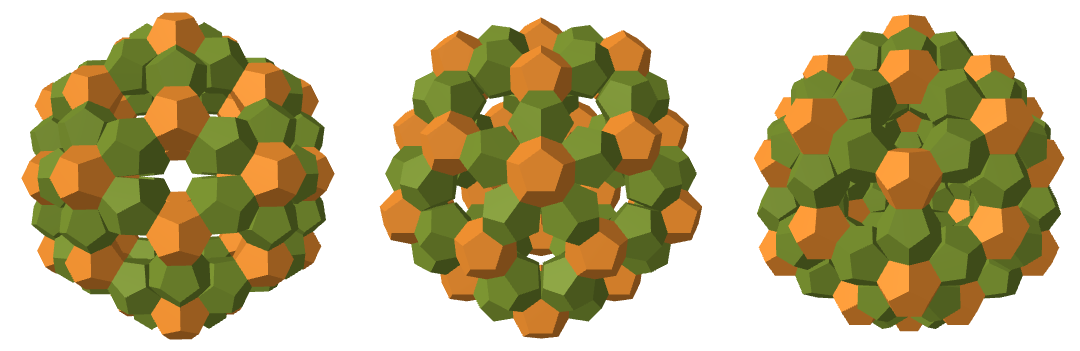
and

(3) A rhombic dodecahedron of truncated octahedra

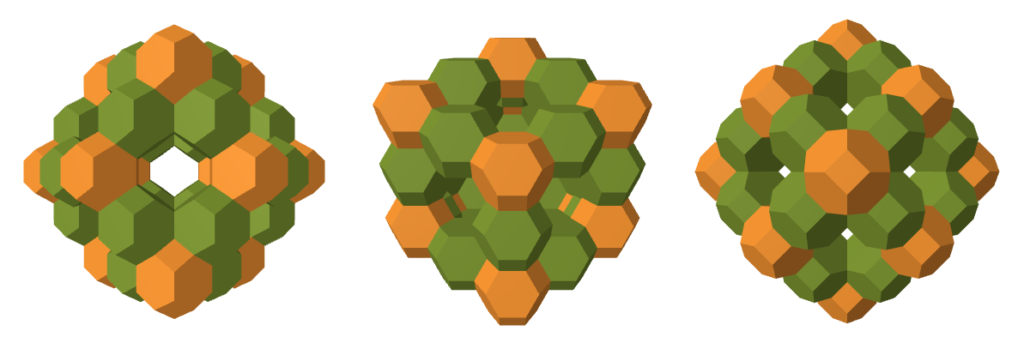
It also turns out that these can be made “in reverse”:

(2*) A regular dodecahedron can be made from rhombic triacontahedra

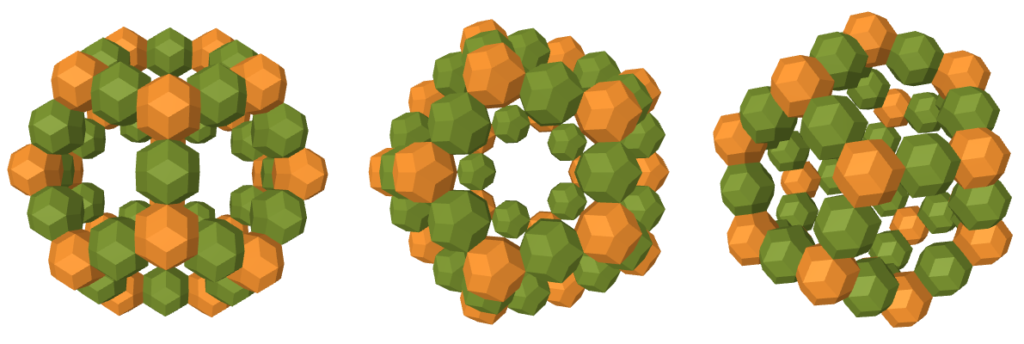
and

(3*) A truncated octahedron can be made from rhombic dodecahedra

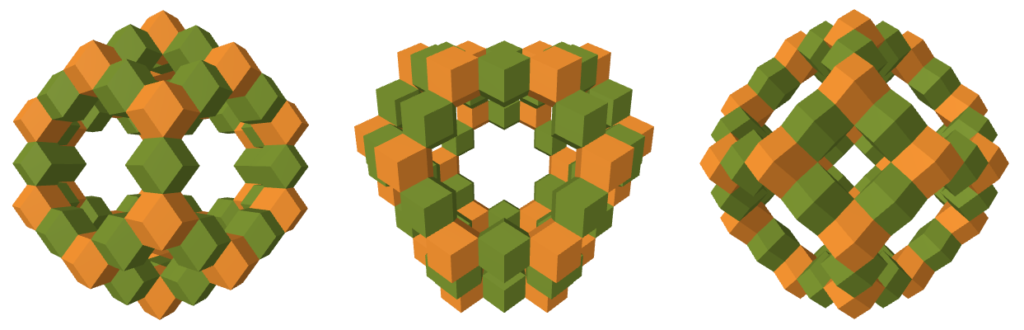
Based on these examples, another natural question is, “When can A be made from B and B also be made from A?

One gets an immediate suspicion that there might be some sort of “skeleton duality” relationship, for example with the cube “skeleton dual” to itself. However, formalizing such a relationship of skeleton duality would require some care in definition to satisfy the properties we like to see in a “duality,” since it is also the case that

(4) A rhombic dodecahedron can be built from regular octahedra

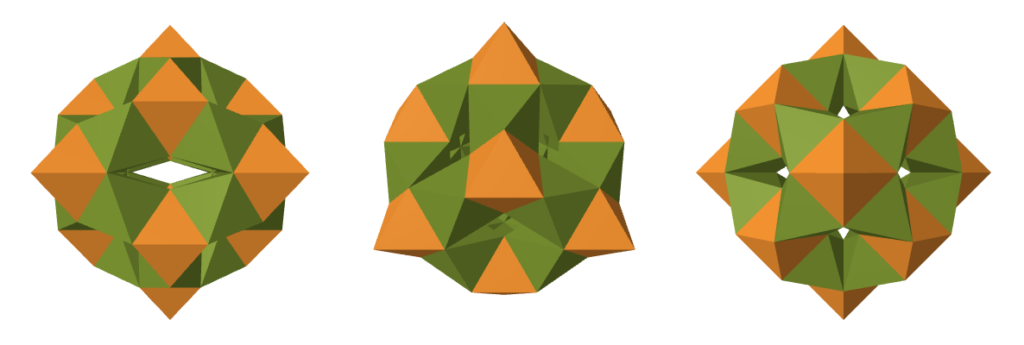
and

(4*) A regular octahedron from rhombic dodecahedra

So the rhombic dodecahedron shares this “can build and can be built from” relationship with both the regular octahedron and the truncated octahedron.

While this relationship is still a little mysterious, we’ll sketch out some ideas about when it exists and how to find it in the coming months.